\noindent The Hamiltonian is:
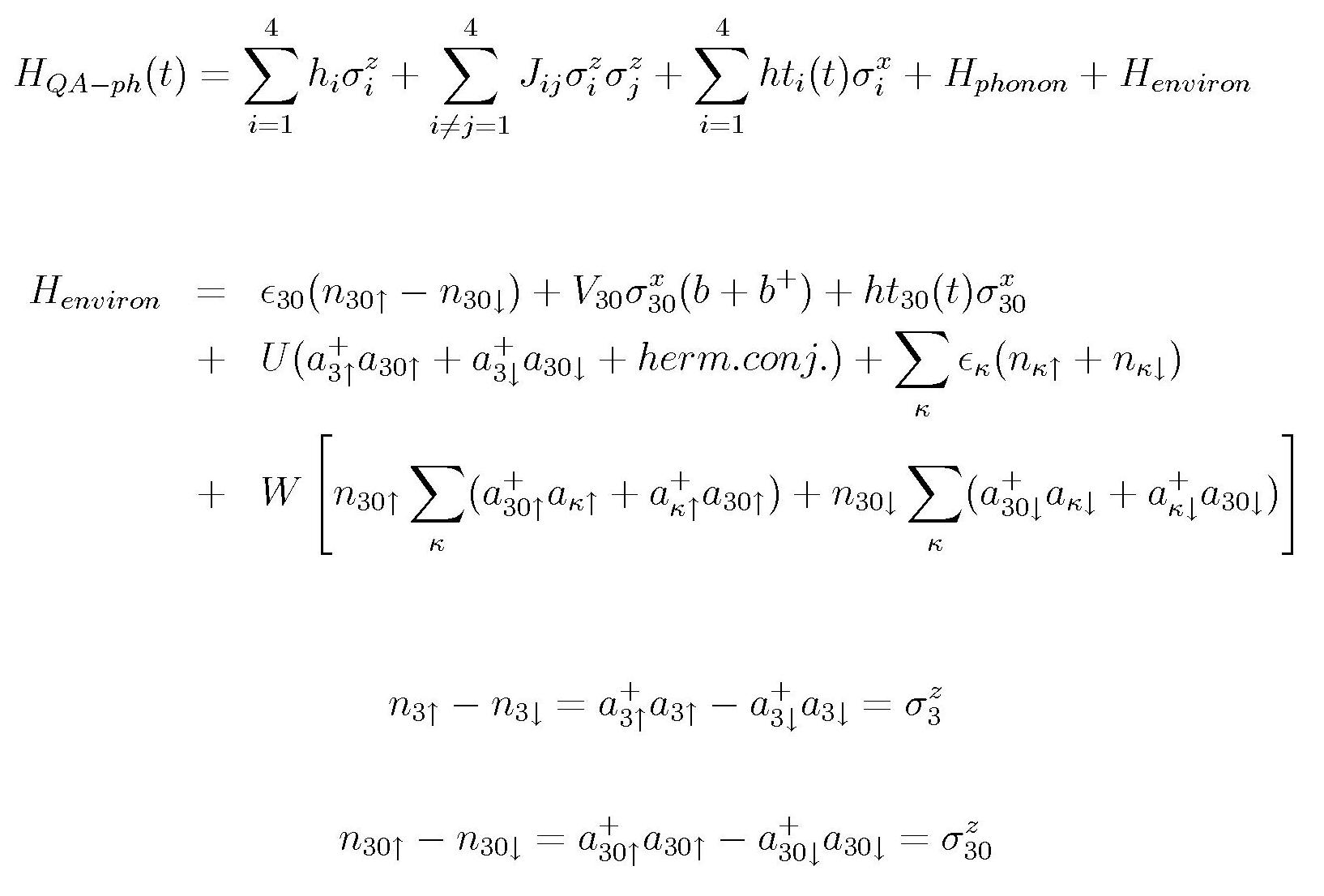
The additional degrees of freedom for the third qubit and the environs have to be
introduced as additional components in the many-particle basis states:
using the notation |i> for the states of the third qubit and |\kappa> for the excited
environmental states. i assumes the values 0,1,30\uparrow ,30\downarrow. \kappa
assumes the values 0,1,2,3,4,5,6,... \kappa =0 represents the situation where
no environmental modes are excited.
The number of basis states increases considerably. We have now four states for the
third qubit. The total number of configurational states is 64\times number of
environmental states. One observes, however, that configurations like e.g. |1111,0,\kappa>
and |1101,0,\kappa> for \kappa >0 do not couple to states with \kappa =0. The
number of configurations to be included in the calculation is therefore 32\times
(1+number of environmental states). If e.g. we would just consider 29 environmental
modes, the number of configurations would be 960. At each time step a 960X960-matrix
has then to be diagonalized.